Part C Image Added Image Added
A person is trying to lift a 10 kg box by applying a perfectly vertical force of 20 N with the help of a pulley. What is the magnitude of the normal force exerted on the box by the floor? Solution System: Interactions: | Cloak |
|---|
| External influences from the earth (gravity), the floor (normal force) and the rope (tension). |
Model: Approach: Diagrammatic Representation We begin with a free body diagram for the box: 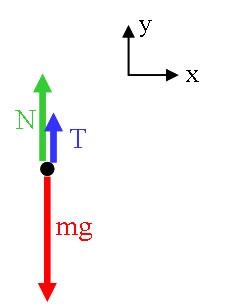 Image Added Image Added
Mathematical Representation From the free body diagram, we can write the equations of Newton's 2nd Law. We ignore the x-direction, since there are no forces acting. | Latex |
|---|
\begin{large}\[ \sum F_{y} = T + N - mg = ma_{y}\]\end{large} |
| 






