{toggle-cloak:id=diaga1} {color:red} *Diagrammatic Representation* {color}

{cloak:id=diaga1}

The ball will translate and rotate as it rolls down the slope.  The relevant free body diagram is shown below.  

!ballonrampfbd.jpg!
{cloak:diaga1}

{toggle-cloak:id=matha1} {color:red} *Mathematical Representation* {color}

{cloak:id=matha1}
From this free body diagram we can construct Newton's 2nd Law for the ball's center of mass and also the angular version of Newton's 2nd Law for rotations of the ball about the center of mass.  The x-component of Newton's 2nd Law is:

{latex}\begin{large}\[\sum F_{x} = mg \sin\theta - F_{f} = ma_{x} \] \end{large}{latex}

and the sum of torques about the center of mass is:

{latex}\begin{large}\[ \sum \tau = RF_{f} = I\alpha \] \end{large}{latex}

{warning}It is not appropriate to assume that the friction force is equal to μ_F_~N~.  The ball is moving, but the point of contact with the ground will remain stationary, and hence we are in the static friction regime.{warning}

We can eliminate the friction force from the two equations to find:

{latex}\begin{large}\[ mg \sin \theta = ma_{x} + I\frac{\alpha}{R}\] \end{large}{latex}

Now, because the ball is rolling without slipping, we can relate the angular acceleration to the linear acceleration of the center of mass:

{latex}\begin{large} \[ \alpha R = a_{x} \] \end{large}{latex}

Substituting this expression allows us to express the acceleration as:

{latex}\begin{large}\[ a = \frac{g \sin\theta}{1 + \frac{\displaystyle I}{\displaystyle mR^{2}}} \]\end{large}{latex}

Using the result that the [moment of inertia] for a sphere is 2/5 m R{color:black}^2^{color}, we have:

{latex}\begin{large} \[ a =\frac{g \sin\theta}{1+\frac{\displaystyle 2}{\displaystyle 5}} = \frac{5 g \sin(30^{\circ})}{7} = \mbox{3.5 m/s} \]\end{large}{latex}

{note}Note that we have been given _no information_ whatever about the mass or radius of the ball!  The acceleration is independent of the mass and the radius.{note}

{cloak:matha1}
{cloak:appa1}
{card:Method 1}
{card:label=Method 2: Angular Momentum}

h4. Method 2: Angular Momentum

{toggle-cloak:id=sysa2} *System:*  {cloak:id=sysa2}The ball as a [rigid body].

{toggle-cloak:id=diaga2} {color:red} *Diagrammatic Representation* {color}

{cloak:id=diaga2}

 Instead of writing separate Laws for the translation and rotation, we could instead choose to simply consider the movement as angular momentum about a fixed axis.  Since the friction force is unknown, it is best to choose an axis on the surface of the ramp such as the one shown in the picture below.

!ballonramp2.jpg!

{cloak:diaga2}

{toggle-cloak:id=matha2} {color:red} *Mathematical Represenation* {color}

{cloak:id=matha2}
The torques about this axis will depend upon the position of the ball.  When the ball has moved a distance _x_ along the ramp, the torques from gravity and the normal force will be:

{latex}\begin{large}\[\sum \tau = mg(x\cos\theta+R \sin \theta) - Nx \] \end{large}{latex}

{note}The expression for the torque due to the ball's weight is most easily found using the lever arm.
!ballonrampfbd2.jpg!{note}


But, using the fact that the ball is not tranlating in the y-direction, we can derive the standard inclined plane relation:

{latex}\begin{large}\[ N = mg\cos\theta\]\end{large}{latex}

which gives:

{latex}\begin{large}\[ \sum \tau = mgR \sin \theta \] \end{large}{latex}

We must now find an expression for the angular momentum of the ball.  Even though we have not chosen to write separate dynamical equations for the rotation about the center of mass and the translation, the ball's angular momentum will still be composed of these two parts:

{latex} \begin{large}\[ L = I_{\rm cm}\omega_{\rm cm} + \vec{r}_{\rm cm,axis}\times m \vec{v}_{\rm cm}= I_{\rm cm}\omega_{\rm cm} + m v_{\rm cm} R \]\end{large}{latex}

Because the ball rolls without slipping, we can relate the angular speed about the center of mass axis to the translational speed of the center of mass:

{latex}\begin{large} \[ \omega_{\rm cm} R = v_{\rm cm} \] \end{large}{latex}

Substituting gives:

{latex} \begin{large} \[ L = I_{\rm cm} \frac{v_{\rm cm}}{R} + mv_{\rm cm}R \] \end{large}{latex}

We now set the time derivative of the angular momentum equal to the sum of the torques, giving:

{latex}\begin{large} \[ \left(\frac{I_{\rm cm}}{R} + mR\right)a = mgR\sin\theta \] \end{large}{latex}

so that we find:

{latex}\begin{large}\[ a = \frac{g\sin\theta}{1 + \frac{\displaystyle I}{\displaystyle mR^{2}}} \] \end{large}{latex}

{cloak:matha2}
{cloak:appa2}
{card:Method 2}
{deck:littledeck}
{card:Part A}
{card:label=Part B}

h3. Part B

Assuming the ball is released from rest, what is the speed of the ball's center of mass after it has moved 1.3 m along the ramp?

h4. Solution

Once again, we solve the problem using two different methods.

{deck:id=littledeck2}
{card:label=Method 1: Kinematics}

h4. Method 1: Kinematics

{toggle-cloak:id=sysb1} *System, Interactions and Models:* {cloak:id=sysb1} As in Part A, plus [One-Dimensional Motion with Constant Acceleration|1-D Motion (Constant Acceleration)].

 
After using one of the methods described in Part A to determine the acceleration, we can use kinematics to find the speed.  The most direct approach is to use:

{latex}\begin{large}\[ v_{x}^{2} = v_{x,{\rm i}}^{2} + 2 a_{x} (x-x_{\rm i}) \]\end{large}{latex}

where we choose _x_~i~ = 0 m.  Solving gives:

{latex}\begin{large}\[ v_{x} = \pm \sqrt{2a_{x}x } = \pm \sqrt{\frac{2gx\sin\theta}{1+\frac{\displaystyle I}{\displaystyle mR^{2}}}} \] \end{large}{latex}

we choose the plus sign, since the ball is translating in the + _x_ direction.  Since the y-velocity is zero, the total speed of the ball's center of mass is:

{latex}\begin{large}\[ v = \mbox{3.0 m/s} \] \end{large}{latex}

Another way to solve the problem is to use energy.  It turns out that in this problem, the mechanical energy of the ball will be constant.  This assertion requires justification.  The forces present in the system are gravity, normal force and friction.  Gravity is a conservative force.  The normal force is non-conservative, but it does no work because it is perpendicular to the motion of the object.  Friction, however, is both non-conservative and directed anti-parallel to the motion of the ball, and so it should clearly do work.  The reason we can assume the energy is constant is the problem's statement that the ball rolls without slipping.  This means that the friction is static rather than kinetic.  Kinetic friction converts mechanical energy into thermal energy and so it is not appropriate to use conservation of mechanical energy when kinetic friction is present.  The work done by static friction, however, does not convert mechanical energy into thermal energy.  Instead, the static friction acts to divert some of the lost potential energy into rotational kinetic energy (rather than simply translational kinetic energy).  Thus, all of the energy remains in a mechanical form.

With this realization, we can write the equation of mechanical energy conservation in the form:

{latex}\begin{large}\[ K_{\rm f} + K_{\rm rot,f} + U_{\rm g,f} = K_{\rm i} + K_{\rm rot,i} + U_{\rm g,i} \]\end{large}{latex}

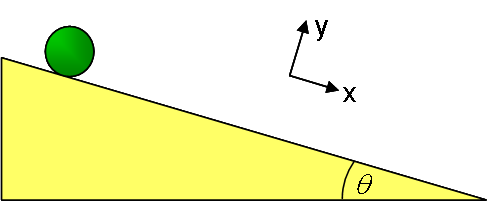
If we select _h_ = 0 at the point of release of the ball, then by the time the ball has moved a distance _x_ along the ramp, it has reached a height:

{latex} \begin{large}\[ h = - x \sin\theta\]\end{large}{latex}

Substituting zeros and appropriate expressions into the conservation of energy formula gives:

{latex}\begin{large}\[ \frac{1}{2}mv_{f}^{2}+ \frac{1}{2}I\omega_{f}^{2} - mgx\sin\theta = 0 \] \end{large}{latex}

Finally, the assumption that the ball is rolling without slipping implies the relationship:

{latex}\begin{large}\[ \omega_{f} R = v_{f}\]\end{large}{latex}

so:

{latex}\begin{large} \[ v_{f} = \sqrt{\frac{2gx\sin\theta}{1+\frac{\displaystyle I}{\displaystyle mR^{2}}}} \] \end{large}{latex}

{tip}The same result as obtained with method 1.{tip}

{info}Looking back at the equations of Part A, method 1 you can see that the friction force is:

{latex}\[ F_{f} = I\alpha /R \] {latex}

We know the value of α from Part A.  Thus, using the fact that the work done by friction when the ball moves a distance _x_ down the ramp is _W_ = -- _xF_~f~ we can write:

{latex}\[ W = - \frac{xI}{R^{2}} \frac{g\sin\theta}{1+\frac{\displaystyle I}{\displaystyle mR^{2}}} \]{latex}

Now, using our result for _v_~f~, we can write this as:

{latex}\[ W = - \frac{1}{2}I \frac{v_{f}^{2}}{R^{2}} = - \frac{1}{2}I\omega_{f}^{2} = - K_{\rm rot} \]{latex}

Thus, the work done by friction is exactly equal to the rotational kinetic energy acquired by the ball.{info}