A person pushes a box of mass 15 kg along a smooth floor by applying a perfectly horizontal force F.  In the process, the 15 kg box pushes against another box with a mass of 10 kg and causes it to move.
  The boxes accelerate horizontally at a rate of 2.0 m/s2.  






Part A



What is the magnitude of F?



Solution



 System: 
 Both boxes together as a single point particle.





 Interactions: 
External influences from the person (applied force) the earth (gravity) and the floor (normal force).



 Model: 
Point Particle Dynamics.



 Approach:  




The word *smooth* in the problem statement is a keyword, telling us that the floor exerts no horizontal force on the box.  Thus, the only external _horizontal_ force acting on the system composed of the two boxes together is that applied by the person. This implies that[Newton's 2nd Law|Newton's Second Law] for the _x_ direction can be written:

{latex}\begin{large}\[ \sum F_{x} = F = m_{\rm system}a_{x} = (\mbox{15 kg} + \mbox{10 kg})(\mbox{2 m/s}^{2}) = \mbox{50 N}\] \end{large}{latex}









Part B



What is the force applied on the front (10 kg) box by the rear (15 kg) box?



Solution



 System:  
This time, we will focus only on the front box, which will be treated as a point particle.





 Interactions: 
The front box is subject to external influences from the rear box, the earth (gravity) and the floor (normal force). 



 Model:  
Point Particle Dynamics



 Approach:  




For simplicity, we will refer to the front box as box 2 and the rear box as box 1.  We will call the force applied by box 1 to box 2 _F_~21~.  The only horizontal force experienced by the front box is the force _F_~21~ provided by the rear box.  Thus, [Newton's 2nd Law|Newton's Second Law] for the _x_ direction can be written:

{latex}\begin{large}\[ \sum F_{x} = F_{21} = m_{2}a_{x} = (\mbox{10 kg})(\mbox{2 m/s}^{2}) = \mbox{20 N}\] \end{large}{latex}









Part C



Suppose we now consider the rear (15 kg) box as an independent system.  How can we reconcile the fact that the force applied by the person to this box is 50 N, but the 15 kg box only accelerates at a rate of 2 m/s2?



Solution



 System: 
The 15 kg box as a point particle.

 



 Interactions: 
The rear box is subject to external influences from the person, the rear box, the earth (gravity) and the floor (normal force). 



 Model: 
Point Particle Dynamics.



 Approach:




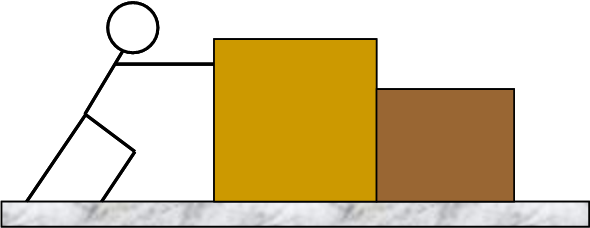
If the 50 N force applied by the person were the only force acting on the rear box, then the acceleration of the box would be 50 N / 15 kg = 3.3 m/s{^}2{^}, but we know that the box is accelerating at 2 m/s{^}2{^}.  To reconcile this apparent contradiction, we must remember that the rear box is not only being pushed on by the person, but also by the front box.  If we again label the rear box as box 1 and the front box as box 2, we can call the force from the front box on the rear box _F_~12~.  It is clear from the relative positions of the boxes that the force from the front box on the rear box would point to the left in the picture above.  Thus, we can write the x-component of Newton's 2nd Law for the rear box in the form:
{latex}\begin{large}\[ F - F_{12} = m_{1}a \]\end{large}{latex}
We can solve this for _F_~12~ to give:
{latex}\begin{large}\[ F_{12} = F - m_{1} a = \mbox{50 N} - (\mbox{15 kg})(\mbox{2 m/s}^{2}) = 20 N \]\end{large}{latex}

{tip}This is equal in size (and opposite in direction) to the force _F_~21~ found in Part B, as is required by [Newton's 3rd Law|Newton's Third Law].{tip}