(Photo courtesy Wikimedia Commons, uploaded by user Che010.)
Bungee cords designed to U.S. Military specifications (DoD standard MIL-C-5651D, available at http://dodssp.daps.dla.mil) are characterized by a force constant times unstretched length in the range kL ~ 800-1500 N. Jumpers using these cords intertwine three to five cords to make a thick rope that is strong enough to withstand the forces of the jump. Suppose that you are designing a bungee jump off of a bridge that is 30.0 m above the surface of a river running below. To get an idea for the maximum cord length, calculate the unstretched length of cord with kL = 4000 N (a system of five 800 N cords) that will result in a 118 kg jumper who leaves the bridge from rest ending their jump 2.0 m above the water's surface. (The 2.0 m builds in a safety margin for the height of the jumper, so you can neglect the height of the jumper in your calculation.) Since you are finding a maximum cord length, ignore any losses due to air resistance or dissipation in the cord. Ignore the mass of the rope.
Solution
System:
Interactions:
Model:
Approach:
Diagrammatic Representation
We begin with an initial-state final-state diagram for this situation, along with corresponding energy bar graphs.
Initial State |
Final State |
|---|
Mathematical Representation
As indicated in the picture, we have chosen the zero point of the height to be the river's surface. With these pictures in mind, we can set up the Law of Change for our model:
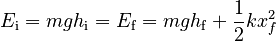
Bungee cords provide a restoring force when stretched, but offer no resistance when "compressed", since they fold like an ordinary rope. Thus, the initial spring energy is zero in this case.
This equation cannot be solved without further constraints, since we do not know k. The extra constraint that we have is given by the fact that the jumper has fallen a total of 28.0 m (descending from 30.0 m above the water down to 2.0 m above the water). This distance must be covered by the stretched cord. This gives us the contraint:

Solving this constraint for xf and substituting into the energy equation gives:
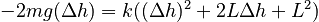
Where we are using Δh = hf - hi to simplify the expression.
We still have two unknowns, but we can resolve this by using the fact that kL is a constant for the rope. Thus, if we multiply both sides by L, we have:

where we have replaced the quantity kL by C (= 800 N) for clarity. With this substitution, it can be seen that we have a quadratic equation in L which can be solved to find:
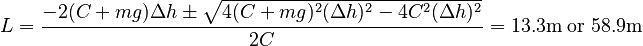
It is clear that the appropriate choice is 13.3 m.
Does the other root have a physical meaning?
A Rule of Thumb
According to bungee jumping enthusiasts, a good rule of thumb is to expect that the maximum length reached by a bungee cord during a jump will be 210% of its unstretched length (assuming the cord is rated for the weight of the person jumping). Thus, a 10 m cord should stretch to 21 m during a jump. How does this rule of thumb compare to our calculated estimate?
Follow Up – Checking Assumptions
In our constraint that:

we neglected the fact that the jumper's center of mass might drop an extra meter or more (depending upon the point of attachment of the cord to the person). What effect would such an extra drop have on the final height?
Follow Up – Checking Assumptions
Suppose a 118 kg jumper foiled your calculations by leaping upward from the bridge with an initial speed of 2.25 m/s. Will the jumper hit the water, assuming the 13.3 m cord with kL = 4000 N that we found in Part A?


