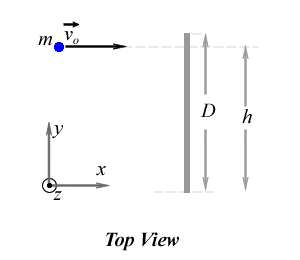
A uniform bar of mass M and length d is initially at rest on a horizontal and frictionless table. A ball of mass m and very small radius is moving towards the bar with speed vo, hits and sticks to the bar at a distance h measured from on end of the bar. The bar is not attached to the table and its moment of inertia with respect to its center of mass is known to be Icm. The goal of the problem is to find the angular velocity and the velocity of the bar's center of mass.
.
Solution:
System: The ball is treated as a point mass and the bar as a rigid body with its mass uniformly distributed. The external forces on the system are the result of the interactions between the elements of the system and the Earth (gravitational force on ball and on bar) and with the table's surface (as there is no fricition it is the normal force exerted by the surface on the ball and on the bar).
Model: 1-D Angular Momentum and Angular Impulse and Linear Momentum and Impulse.
Approach:
The motion of the objects before and after the collision is on the table's surface, _(x,y)_ plane. After the collision, the ball-bar system will translate to the right and rotate counter-clockwise about its center of mass which is not at the center of the bar but at some point between the ball and the center of the bar.
To solve this problem we apply linera momentum and impulse and angular momentum and angular impulse principles to the ball-bar system. For that purpose we need to calculate the sum of the external forces exerted on the system and the sum of the torques due to the external forces about a given point.
The sum of the external forces:
The external forces on the system are the gravitational and the normal force applied at the center of mass of ball and the bar. Because there is no motion in the direction perpendicular to the table's surface (z-axis), then the normal force on both objects has the same magnitude and opposite direction than the gravitational force. Therefore, the sum of the external forces on the system is zero and the linear momentum of the system is constant:
$\vec P_
= \vec P_
$
![]()
The sum of the external torques about a given point Q:
The net torque due to the external forces about a given point Q is:
$\sum \tau_
^
= \tau_
^
+\tau_
^{N_{ball}} + \tau_
^
+\tau_
^{N_{bar}}$
We chose an arbitratry point Q on the table and we measured the position of the ball's center of mass with the vector
$\vec r$
, red vector in the figure. The normal force exerted on the ball,
$\vec N_
$
, and the gravitational force on the ball,
$\vec F_
$
are indicated in black in the figure. Because these two forces are applied at the same point, the ball's center of mass, then the moment arm of these two forces are the same. In addition, these forces have the same magnitude and opposite direction, therefore the torques due to each of these forces has the same magnitude and opposite direction and they will add to zero. The torques are indicated with the purple vectors in the figure.
$ \tau_
^
+\tau_
^{N_{ball}}=\vec r x \vec F_
+ \vec r x N_
= 0$
.
![]()
In the same way, we can calculate the torques due to the normal and the gravitational force exerted on the bar. These two forces are also applied at the same point, the centre of mass of the bar, therefore the moment arm from point Q is the same and because these two forces have the same magnitude and opposite direction the resulting torques due to these to forces about point Q will add to zero. We conclude that the sum of the torques due to the external forces on the system about point Q is zero, therefore that angular momentum of the ball-bar system about point Q is constant. In this particular problem the point Q can be any point so the torque will be zero about any point and the angular momentum will be constant about any given point.