Taylor Columns
1 Intro
One of the most interesting properties of a homogenous fluid in geostrophic flow is its ‘rigidity’-- in both the horizontal and vertical direction, the velocity vector does not vary in the direction of rotation. This is known as the Taylor-Proudman theorem, which explains the appearance of Taylor Columns, the subject of our project.
A Taylor column appears when an object (such as a hockey puck) is placed in a homogenous, flowing liquid; the resulting flow of fluid past that object will obey the Taylor-Proudman theorem, meaning that the vertical columns of water will move along contours around the object at all depths, including above and below the object. A diagram of this occurance is depicted below in Figure 1.
Figure 1. The object at the bottom creates a stationary column of fluid that extends to the surface of the fluid. This is a Taylor column.
There are a number of stated conditions needed for Taylor columns to exist; they will only form in a slow, steady, frictionless flow of a homogenous fluid above a rather uniform shape. Evidently, in the ocean and atmosphere, there is always going to be varying densities, friction, and disturbances. As a result, ‘proper’ Taylor columns, such as the ones that can be created in a rotating fluid lab setting, are very rare. Despite this, Taylor columns, the Taylor-Proudman theorem have very real applications in the world because of its relation to thermal wind, which we will discuss later.
Below is a photo of an island in the Galapagos taken by a satellite. The island blocks the flow of air on the surface layer, forming a Taylor column that can be seen as the gap in the clouds.
Figure 2. A satellite photo of the Galapagos islands. Here, air is flowing from roughly left to right. The island, the “object”, is situated in the bottom left hand corner. The clouds, tracers in this case, clearly show that above the island, a column extends, breading the flow of the clouds. Behind the Taylor column, in the swirling motions, is the wake caused by the islands Taylor column.
2 Theory (The Taylor-Proudman Theorem)
The Taylor-Proudman Theorem says that for a fluid meeting the conditions stated above, velocity will not vary in vertical direction. To derive this conclusion, we will start with the momentum equation of a fluid in geostrophic balance with negligible friction where the Coriolis force, pressure gradient, and gravity are balanced, which is
2Ω x u + (1/ρ)∇p + ∇φ = 0 (1)
Where Ω is the rotation vector, u is the flow vector, p is the pressure and φ is the gravitational potential modified by centrifugal accelerations.
When ρ = ρ(p), meaning that density is a function of the pressure, taking the curl of this equation yields the following:
(Ω⋅∇)u = 0 (2)
because the curl of a gradient is 0.
Ω acts in the virtical direction, therefore this equation reduces to,
∂u/∂z = 0 (3)
The implication of this equation is that velocity does not vary with height; the fluid forms vertical columns that are not disrupted. These vertical columns are called Taylor columns. According to the assumptions we made to derive this equation, in order for Taylor columns to form, the fluid must be barotropic (ρ = ρ(p)), inviscid (frictionless), and in geostrophic flow (Ro << 1). Because these conditions are rarely met in nature, Taylor columns do not usually occur in natural systems.
A simpler result can be shown by taking the geostrophic balance equation:
fzxu + 1/ρ∇p (4)
Solving for u yields the following:
u = 1/fρz x ∇p (5)
Or, in component form:
(u, v) = (-1/fρ∂p/∂y, 1/(fρ)∂p/∂x) (6)
Taking the derivative w/ respect to z yields the following:
(∂u/∂z, ∂v/∂z) = (-1/(fρ)∂/∂y∂p/∂z, 1/(fρ)∂/∂x∂p/∂z) (7)
By hydrostatic balance, ∂p/∂z can be rewritten as -ρg, and if ρ and f are constant, then their derivatives with respect to position are zero. Therefore, the horizontal wind does not vary with height.
3 Experiment
There were two parts to our experiment: the first was to test whether we could replicate Taylor columns with an object placed in a rotating tank and the second was to test whether there would still be a disruption when an object was placed in a tank with water that had a temperature gradient
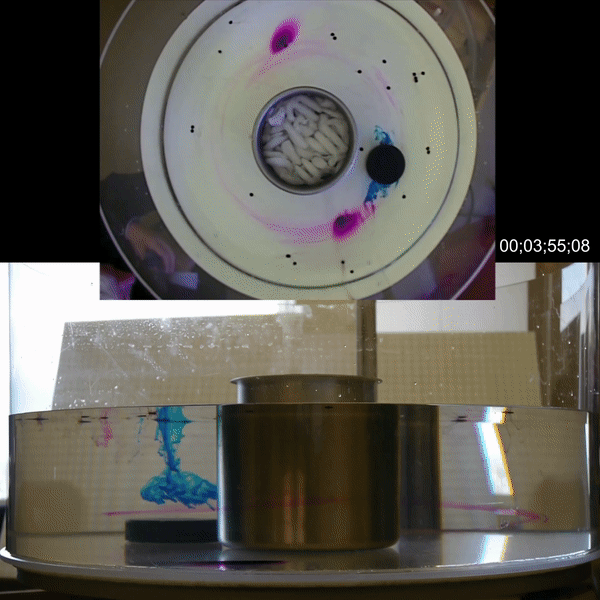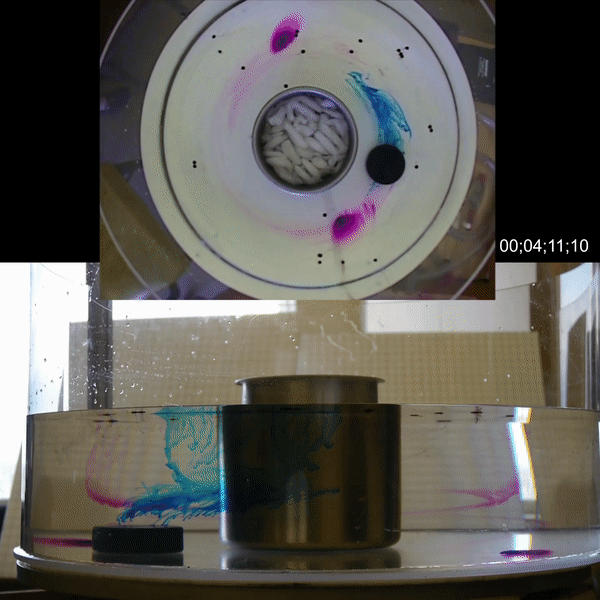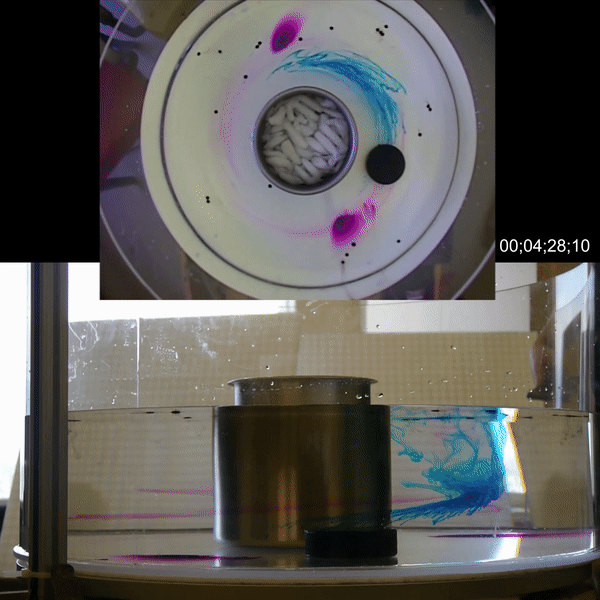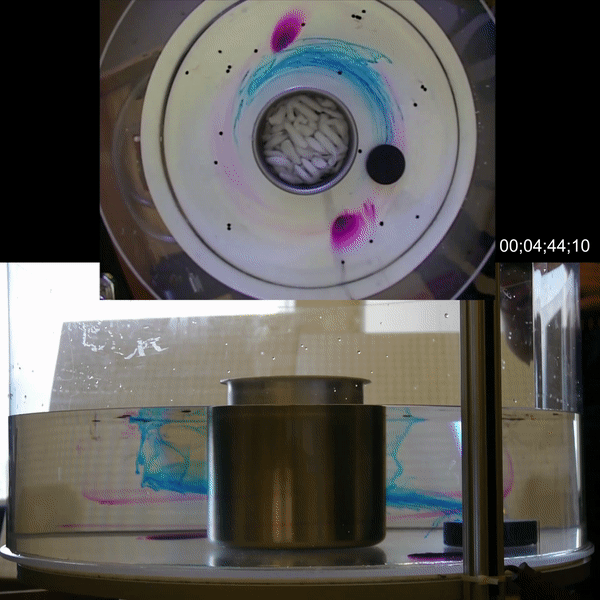
For the first experiment, we filled a cylindrical tank with water and placed it on a rotating turntable. At the bottom of the tank, we placed an object the size of a hockey puck. The tank was spun up to ~4rpm and once it was in solid body rotation, we decreased the rpm very slightly, by ~0.5rpm. Slowing down the rotation meant that until the water re-entered solid body rotation, it was rotating in the tank’s frame of reference. As explained in the theory, velocity should not vary with height, so when the water at the bottom of the tank went around the object, all the water above should also go around the object. As shown in Figure 3, we didn’t see the particles or permanganate streaks go completely around the object, like expected from the theory. However, the particles are deflected, so there is some change in the flow of water above the object. The reason why we didn’t see a complete observance of the theory is probably because not all of the conditions for the Taylor-Proudman Theory were met. There was some turbulence in the tank because the turntable was not completely flat, which meant that the water wasn’t steady. Additionally, there was air blowing on the top of the tank because the tank was not isolated, which could have disrupted the surface and led to a density gradient if there was heat transfer between the water and the air.
Figure 3. The results of experiment 1, showing that even though surface particles and permanganate streaks don’t completely avoid the area above the object, their paths are diverted.
Figure 4. The setup of the second experiment with the ice bucket in the middle of the tank.
In our second experiment, we again filled a cylindrical tank with water and placed it on a rotating turntable, but we added a bucket filled with ice to the center of the tank to create a meridional temperature gradient. A cylindrical object was placed in the tank some distance from the axis of rotation. The cold from the ice bucket generated a Hadley circulation and a zonal flow. As with the previous experiment, particles were used to track the surface flow. Due to the horizontal density gradient, the velocity of columns vary vertically, so the Taylor columns become tilted. Figure 5 confirms this variation in vertical velocity as the columns of dye dropped into the tank begin to spiral. However, it does not seem like the object in the tank interfers with the movement of this column.
Figure 5. A video of experiment 2, showing that the density gradient causes the Taylor column to tilt.
4 Thermal Wind
To begin to understand the relationship between Taylor columns and thermal wind, it is important to understand isothermal surfaces, which is a surface where the temperature of the fluid or air is equal everywhere. Isothermal surfaces can be made in both the lab and exist in the atmosphere. These surfaces are, in both rotating fluid labs and in the atmosphere, three dimensional surfaces. Lets first look at an example of a surface visible in a lab.
Figure 6. Isothermal surfaces outlined by green dye in the second experiment. This surface is a conical shape, wide at the bottom and smaller near the surface.
We can think of this isothermal surface as a type of Taylor column because it has similar properties to the Taylor column-- its rigidity and equanimity over the 3-dimensional surface. Why this surface is tilted, as opposed to straight up, has to do with the curl of the Taylor column opposing gravity. In the center of the tank in the above photo is ice, creating a Hadley cell as shown by the arrows. The colder water is on the bottom, and moving out as it is displaced by warmer water cooling and going down (shown by the blue arrow). The spinning motion, geostrophic balance, and inhomogeneous nature of the water here is what makes this surface, this Taylor column, tilt over. The reason why it remains tilted instead of collapsing due to gravity is because the overturning torques of the Taylor column balance the Coriolis and gravitational forces.
Thermal winds happen all over the earth, from scales as small as cool ocean air meeting warmer air above land, to as large as the polar vortex. As winds move around the earth, they carry with them their thermal properties, creating weather. Isothermal and isobaric surfaces, warped and tilted because of landforms, friction, density differences and latitude differences, extend far up into the atmosphere.