You are viewing an old version of this page. View the current version.
Compare with Current
View Page History
« Previous
Version 48
Next »
Unknown macro: {table}
Unknown macro: {tr}
Unknown macro: {td}
Error formatting macro: live-template: java.lang.NullPointerException
Unknown macro: {td}
One way that we perceive weight is the normal force we experience from the ground. In physics problems, when you are asked to determine apparent weight, the quickest method is usually to compute the normal force provided by the "ground".
One way to experience a reduced apparent weight is to strap into a harness of ropes and have someone (or some weight) pull down on the other end like they do in theater or films. Another way is to jump into a swimming pool, where the water lifts up on you. Another possibility, which we explore in this problem, is to enter an environment where the "ground" is capable of moving, such as an elevator.
Part A
Suppose a person with a weight of 686 N is in an elevator which is accelerating downwards at a rate of 3.0 m/s2. What is the person's apparent weight?
Solution
System:
Person as a point particle.
Interactions:
External influences from the earth (gravity) and the floor of the elevator (normal force).
Model:
Point Particle Dynamics.
Approach:
Diagrammatic Representations
The physical picture and free body diagram for the person is:

|
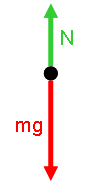
|
Physical Picture |
Free Body Diagram |
Mathematical Representation
which leads to the form of Newton's 2nd Law for the y direction:
Unknown macro: {latex}
\begin
Unknown macro: {large}
[ \sum F_
Unknown macro: {y} = N - mg = ma_
]\end
In our coordinates, the acceleration of the person is ay = -3.0 m/s2, giving:
Unknown macro: {latex}
\begin
Unknown macro: {large}
[ N = ma_
Unknown macro: {y}
+ mg = \mbox
Unknown macro: {476 N}
]\end
Is the answer sensible?
Is it clear why the acceleration must have a minus sign?
Part B
Suppose a person with a weight of 686 N is in an elevator which has been ascending at a constant rate of 1.0 m/s and is now slowing down at a rate of 3.0 m/s2. What is the person's apparent weight?
Solution
System, Interactions and Model:
As in Part A.
Approach:
As in Part A, the acceleration is negative in our coordinates. (Why?) The free body diagram is also the same, and so we find the same result:
Unknown macro: {latex}
\begin
Unknown macro: {large}
[ N = \mbox
Unknown macro: {476 N}
]\end
Part C
Suppose a person with a weight of 686 N is in an elevator which is ascending, speeding up at a rate of 3.0 m/s2. What is the person's apparent weight?
Solution
System, Interactions and Model:
As in Part A.
Approach:
The free body diagram and form of Newton's 2nd Law is the same as in Part A, except that the relative size of the forces will be different. We can see this by writing Newton's 2nd Law for the y-direction:
Unknown macro: {latex}
\begin
Unknown macro: {large}
[ N = ma_
Unknown macro: {y}
+ mg ]\end
This time, however, the acceleration is positive (Why is this?) Notice that the elevator's velocity does not affect the result, and does not appear in the formula. The acceleration is (ay = + 3.0 m/s2) giving:
Unknown macro: {latex}
\begin
Unknown macro: {large}
[ N = \mbox
Unknown macro: {896 N}
] \end